u-sub Archives | Jake's Math Lessons

Before reading through this problem, I’d recommend checking out my lesson on finding volumes of rotation using the cylinder shell method. I’m not going to go into quite as much detail here as I did in that lesson. It might help you make more sense of what’s going on if your start there.

Other than that there isn’t much else to add so let’s jump into an example!

Example 1

Find the area of the solid created by rotating the area bounded between $y= (x-1)^3-3$ , $y=-x-2$ , and $y=-2$ about the line $y=-1$ .

Just as before I’ll use the same 4 step process as in the cylinder method lesson.

1. Graph the 2-D functions

As I always say, I suggest starting any problem possible by drawing what is being described to you. Go ahead and start with graphing all of the functions described in the problem. I’ll do this using Desmos. You should end up with something like the graph below. I also went ahead and shaded the bounded region gray to make it a little easier to see (this was not done in Desmos).

$y= (x-1)^3-3$ , $y=-x-2$ , $y=-2$ , and $y=-1$

$y= (x-1)^3-3$ , $y=-x-2$ , $y=-2$ , and $y=-1$

2. Rotate the 2-D area around the given axis

Again, we want to visualize what the question is asking us to find. We will need to take the shaded region in the above graph and rotate it around the line $y=-1$ . Doing this would create a 3-D figure whose volume we’ll need to find. But first let’s draw it.

To do this, imagine the 2-D gray region coming off the paper or screen and rotating around the axis of rotation. Doing this would give us something like the figure below.

Figure resulting from rotating the area around a horizontal. — Result of rotating the region about the line $y=-1$ .

Result of rotating the region about the line $y=-1$ .

3. Setting up the integral

I’m not going to go into as much detail to explain where this integral comes from as I did in the cylinder method lesson, but if the following integral confuses you I’d recommend checking that lesson out by clicking on the above link.

Long story short, we want to imagine our 3-D figure is made up of several infinitely thin cylindrical shells. Adding up the volume of all of these shells would result in an integral like this: $$\int 2 \pi r h \ dr.$$

In order to help with coming up with each of these pieces, we need to relate them back to our figure and the functions that created it. In order to visualize this, let’s draw our figure with one of these infinitely thin shells that make up the entire figure. We can consider this one shell and how to represent these dimensions in terms of the given functions.

You can see one of these cylindrical shells represented in the drawing below with a labeled version of the cylinder draw in the upper-right hand corner.

3-D figure with a cylindrical shell — 3-D figure with a sample cylindrical shell shown in green.

As with all cylinder shell method problems, we need to imagine integrating from the center of the cylinder out to the outer edge. Since our cylinder is laying horizontally, moving from its center to its edge moves up and down. This means we are moving in the y direction. Therefore, we need to integrate in the y direction and represent our integral only in terms of y (we shouldn’t have any x‘s).

So let’s think about each of the three pieces that make up our integral one at a time.

Finding r

The radius of this cylinder would simply be the distance between the center of the cylinder and the edge. You can see in the smaller version of the cylinder drawn off to the side that the radius is represented by the red line measuring between the points labeled $(x_2, \ -1)$ and $(x_2, \ y)$ .

Since these two points have the same x value, we can find the distance between them by simply finding the distance between their y values. To do this we just need to take the larger value and subtract the smaller one from it. $$r=-1-y$$

Finding h

The height of a cylinder will always be measured as the distance between the two flat, parallel faces. Usually they would be the top and bottom, but since our cylinder is sideways, we need the distance between the left side and right side.

Looking at the smaller cylindrical shell off to the side in the drawing above, you can see the height of this cylinder is represented by the red line measuring the distance between the points $(x_1, \ y)$ and $(x_2, \ y)$ .

Similar to what we did before, these two points have the same y value. As a result, the distance between them would be the same as the distance between their x values. So we just need to take the larger x value and subtract away the smaller one. $$h=x_2-x_1$$

But remember earlier I said we need everything just in terms of y?

So we need to think about how we can rewrite $x_1$ and $x_2$ in terms of y.

Finding 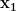$\mathbf{x_1}$

We know that $x_1$ lies on the function $y=-x-2$ so we know that the relationship between $x_1$ and y can be described in the same way $$y=-x_1-2.$$ If we rearrange this to solve for $x_1$ instead of y, we can use this to replace the $\mathbf{x_1}$ in our equation for h. $$y=-x_1-2$$ $$y+x_1=-2$$ $$x_1=-y-2$$

We can use this to rewrite h but replace the $x_1$ with $(-y-2)$ since we know they are equal. $$h= \ x_2- (-y-2)$$ Now we need to do the same thing with $x_2$ .

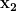
Finding $\mathbf{x_2}$

We are going to apply the same idea here as in the previous section. We know that $x_2$ lies on the function $y= (x-1)^3-3$ . Therefore, we can describe the relationship between $x_2$ and y as $$y= (x_2-1)^3-3.$$ Now we can solve this equation for $x_2$ and plug this into our equation for h. $$y \ = \ (x_2-1)^3-3$$ $$y+3 \ = \ (x_2-1)^3$$ $$\sqrt[3] {y+3} \ = \ x_2-1$$ $$\sqrt[3] {y+3} +1 \ = \ x_2$$ Now going back to our equation for h, this tells us $$h \ = \ \sqrt[3] {y+3} +1 – (-y-2).$$ And to simplify a bit: $$h \ = \ \sqrt[3] {y+3} +1 + y+2$$ $$h \ = \ \sqrt[3] {y+3} + y+3.$$ Now that we have h and r, we just need to find dr.

Finding dr

This is actually the simplest part to find. The dr represents the change in the cylinder’s radius as we go from each shell to the next. Since we move in the same direction of the radius as we integrate to find our volume, the change in r should be the same as the change in y between each step. Therefore, we can say that $$dr=dy.$$

Putting it all back into an integral

We already figured out that the volume of our figure can be found by using the integral $$\int 2 \pi rh \ dr.$$ And we just found these three pieces to be $$r=-1-y$$ $$h \ = \ \sqrt[3] {y+3} + y+3$$ $$dr=dy.$$ So we can just plug them into our integral. $$ \int 2 \pi \ ( -1-y ) \ \Big( \sqrt[3] {y+3} + y+3 \Big) \ dy$$

Now we need one last piece. We need to add bounds on the integrals.

Since we are integrating with respect to y, the bounds of our integrals need to be the range of y values that make up our original 2-D area. Looking back at our original graph, we can see that the original area bounded by the given functions spans over all of the y values between $y=-2$ and $y=-3$ . Therefore, we know that the volume of our figure will be $$V \ = \int_{-3}^{-2} 2 \pi \ ( -1-y ) \ \Big( \sqrt[3] {y+3} + y+3 \Big) \ dy.$$

4. Solve the integral

Now all we need to do is solve the integral we just found and that will leave us with our volume. This is actually a pretty complicated integral as is it, so let’s start with simplifying it a bit. We’ll do this by pulling out the constant, distributing out through the parenthesis, and combining like terms.

$$V \ = \int_{-3}^{-2} 2 \pi \ ( -1-y ) \ \Big( \sqrt[3] {y+3} + y+3 \Big) \ dy$$ $$V \ = \ 2 \pi \int_{-3}^{-2} \ – \big(y+3 \big)^{\frac{1}{3}} – y \ – 3 -y \big(y+3 \big)^{\frac{1}{3}} – y^2 – 3y \ \ dy$$ $$V \ = \ 2 \pi \int_{-3}^{-2} \ – \big(y+3 \big)^{\frac{1}{3}} -y \big(y+3 \big)^{\frac{1}{3}} – y^2 – 4y -3 \ \ dy$$

Now that we have it in a form that is simplest to integrate we can go ahead and integrate this function one term at a time. I’m not going to show every step of how to do this, but if you’d like to work it out on your own, I’d suggest using u-substitution on the $-(y+3)^{1/3}$ term and using integration by parts on the $-y(y+3)^{1/3}$ term.

$$V \ = \ 2 \pi \Bigg[ – \frac{3}{14} \big( y+3 \big)^{\frac{4}{3}}\big( 2y-1 \big) – \frac{1}{3}y^3 – 2y^2 – 3y \Bigg]_{-3}^{-2}$$

Again, I’m not going to show every step of this. Instead I used Wolfram Alpha from here, but if you evaluate this expression from $y=-3$ to $y=-2$ , you’ll see that $$V \ = \ 2 \pi \bigg(\frac{73}{42} \bigg)$$ $$V \ = \ \frac{73 \pi}{21}$$

Hopefully all of this helps you gain a bit of a better understanding of this method, but as always I’d love to hear your questions if you have any. Just email me at jakesmathlessons@gmail.com and I’ll see if I can help provide a bit more clarification. You can also use the form below to subscribe to my email list and I’ll send you my bonus FREE calc 1 study guide! Just put in your name and email address and I’ll be sure to let you know when I post new content! Feel free to go check out my other lessons and solutions about integrals as well.

U-substitution is one of the more common methods of integration. It allows us to find the anti-derivative of fairly complex functions that simpler tricks wouldn’t help us with. The best way to think of u-substitution is that its job is to undo the chain rule.

That’s all we’re really doing.

It’s not too complicated when you think of it that way. Although, the execution isn’t always that simple. But I’ll show you 6 simple steps that will help you solve any u-substitution problem!

1. Picking our u

A u-substitution problem will start out similarly to an integration by parts problem. With any u-substitution problem the first thing you will need to do is decide what piece of the function you will call u. This is the most important piece of the process, and really the only part where there are options to choose from. However, there’s a simple trick to make sure you’re selecting the u correctly.

When deciding which part of your function to call u, you will want to look for a piece of your function that you can see that piece’s derivative somewhere else in the function. That sounds a little strange, but let me give you an example.

Say we have some function like $f(x)=x(x^2+5)^3$ . We want to look for a small piece of this function that also has its own derivative somewhere else in the function.

So we might say $u=x^2+5$ because the derivative of $x^2+5$ is just 2x. Notice, our function contains an x, not a 2x, but it’s fine if the derivative differs by a constant like this. It’s easy to deal with the constant here, but it’s important that it’s an x term.

A quick note on substitution

Choosing the correct u in these problems is the most challenging part. It’s not always simple to see what the u should be, so it’s important to be willing to try different things and see what happens.

You may end up needing to pick a u to go a few steps into the problem and realize it won’t work, then go back and pick another u. I know this process can be frustrating at times, we’ve all been there, but sometimes trial and error is required in learning new math concepts. So I urge you to stay persistent and keep picking different parts of the function for u.

If you try calling every possible piece of the function u, and work through the next few steps only to find that none of them will work, you likely need to either use some uncommon trick or find the derivative using another method besides u-substitution. I will get into some of the uncommon tricks in a later post.

2. Finding du

Once you have decided which piece of your function will be u, you then need to calculate du. This should be fairly simple.

All you have to do to find du is take the derivative of u then multiply it all by dx. This will sometimes require the use of the chain rule, product rule, or quotient rule, but usually you will just need the power rule.

Let’s think back to our previous example, finding the antiderivative of $y=x(x^2+5)^3$ . Remember, we decided that we would use $u=x^2+5$ . Therefore, to find du we should take it’s derivative and multiply by dx. This means if

$$u=x^2+5, \ then$$

$$du=2x \ dx.$$

3. Solve for dx

Now we have determined which part of our function we will call u and we found du. However, what we really want is to find dx. This will be easier to work with when we do our substitution into the original function.

All we need to do to find dx is take our equation for du and isolate the dx. In this example, this will be very easy.

$$du=2x \ dx$$

$$\frac{du}{2x}=dx$$

4. Substitute back into the original function

Going back to the function we are trying to find the antiderivative of, we will first write this in integral form.

$$\int x(x^2+5)^3 dx$$

Now we just need to substitute our u and dx back into this integral. This requires replacing the $x^2+5$ with u, and replacing dx with $\frac{du}{2x}$ . This gives us

$$\int x(u)^3 \frac{du}{2x}$$

After making these two substitutions we should be able to do some simplifying that will cancel out any remaining x‘s. Simplifying this integral should leave us with

$$\int \frac{1}{2}u^3 du.$$

And since we can pull constants out of an integral, this can also be written as

$$\frac{1}{2} \int u^3 du.$$

5. Integrate with respect to u

Looking at the above integral, we can see that we no longer have an x in the problem. We have rewritten everything in terms of u. Since our integral contains only u and du, instead of x and dx, we can integrate with respect to u. This simply means that we are taking the antiderivative of the function $g(u)=u^3$ where u is our variable.

Notice, this integral is much simpler than the one we started with. All we need to find this one is the power rule for antiderivatives.

$$\frac{1}{2} \int u^3 du$$

$$\frac{1}{2} \cdot \frac{1}{4}u^4$$

$$\frac{1}{8} u^4$$

Now the hard part is over, we found the antiderivative. But unfortunately we aren’t quite done yet. We can’t say that $\frac{1}{8}u^4$ is the antiderivative of $f(x)=x(x^2+5)^3$ . This doesn’t really have any meaning because they are using two different variables. Instead we need to write the answer in terms of x, since that’s what we started with.

6. Substitute x back in

We are almost done now that we found the antiderivative. We just need to write it in terms of x so that our answer actually is the antiderivative of the function we started with. Since we already found the antiderivative in terms of u, and we know u in terms of x, we can simply substitute in for u.

We decided back in step 1 that

$$u=x^2+5$$

and we also found out that our antiderivative in terms of u is

$$\frac{1}{8}u^4.$$

Therefore, we can plug $x^2+5$ in for u to find that the antiderivative of $f(x)=x(x^2+5)^3$ is

$$F(x)= \frac{1}{8}(x^2+5)^4 + c.$$

And that’s it! You can apply these 6 steps to solve any u-substitution problem.

I hope this lesson helps, but if there’s still a topic you’d like to learn about take a look at some of my other lessons and problem solutions. If you can’t find the topic or question you’re looking for just let me know by emailing me at jakesmathlessons@gmail.com!

I also encourage you to join my email list! Just enter your name and email below and I’ll let send you my calc 1 study guide as a bonus to help you survive calculus!

Tag: u-sub

Cylinder/Shell Method – Rotate around a horizontal line